MacBook Pro: perché Apple dice addio alla Touch Bar...
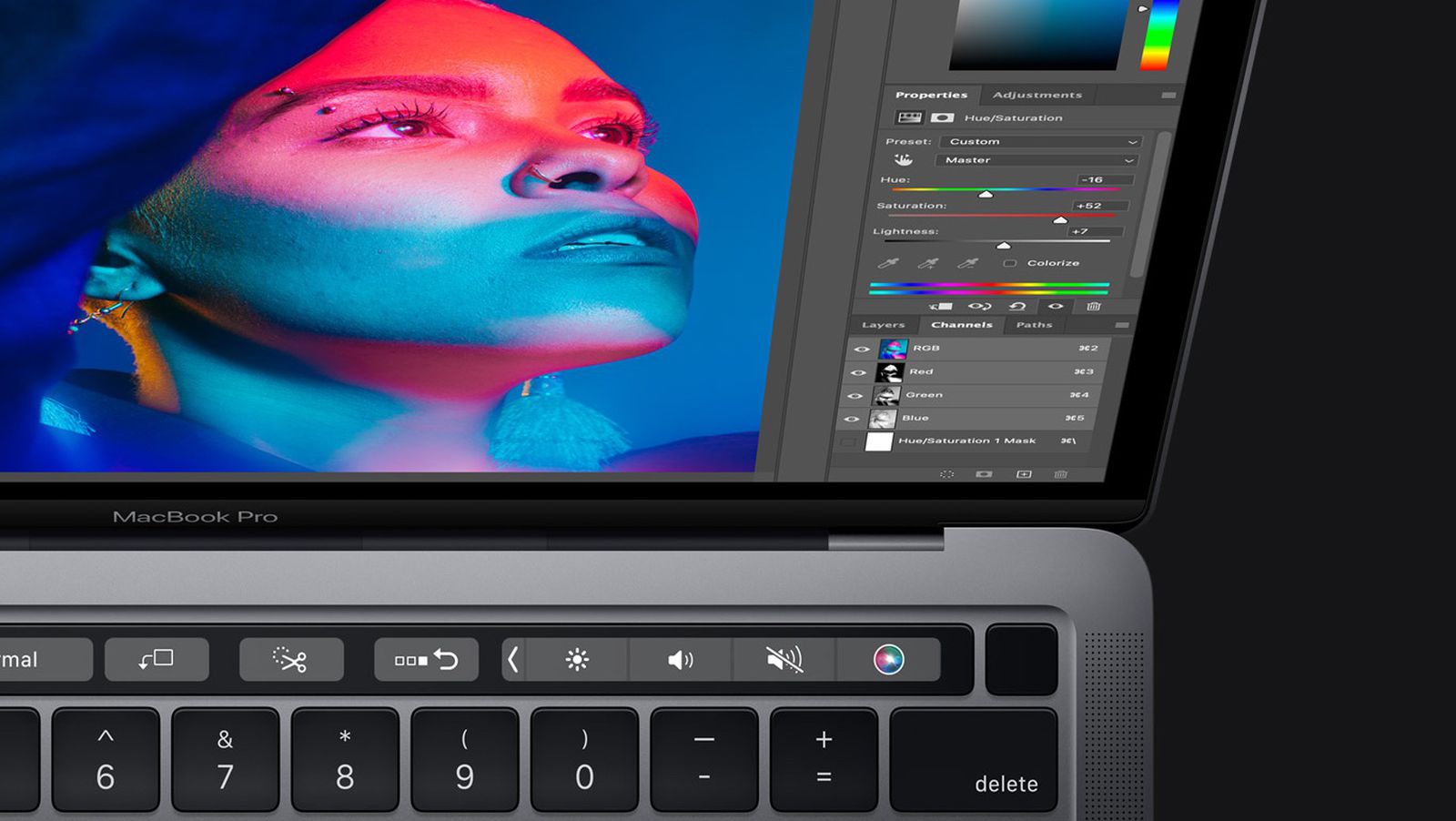
The MacBook Pro Touch Bar gets a second life as this portable, Apple-inspired accessory - Yanko Design
Why Apple Finally Killed the Touch Bar on the MacBook Pro | Digital Trends
Macbook Pro 13" M2 TouchBar 2022 Ricondizionato usato rigenerato e rimesso a nuovo
Apple's MacBook Pro Touch Bar is gone. Good riddance - CNET
Touch Bar: Apple l'ha abbandonata, ma i produttori di PC ci credono ancora - macitynet.it
Apple patents new MacBook's Touch Bar with Force Touch technology - 9to5Mac
Touch Bar su MacBook: cos'è e come funziona | WizBlog
Come eliminare i dati dalla Touch Bar del MacBook Pro - Italiamac News
Apple's next MacBook Pros might dump the Touch Bar, revive MagSafe | Macworld
Touch Bar sì, Touch Bar no: la scintilla che ancora per molti stenta a scoccare
Apple Il Computer Portatile MacBook Pro Touch Bar 16´´ I9 2.3/16GB/1TB SSD Argento| Techinn
Why Apple Removed The MacBook's Touch Bar - YouTube
Come funziona la Touch Bar dei nuovi MacBook Pro in Photoshop.
Touch Bar per Pages sul Mac - Supporto Apple (IT)
Apple No Longer Sells a MacBook Pro Without a Touch Bar - MacRumors
MacBook Pro 13: il nuovo modello (senza Touch Bar) arriverà dopo il WWDC | SmartWorld
Ledge Macbook Pro Touch Bar Lock Adapter
Apple MacBook Pro 2018 | 15.4" | Touch Bar | 2.6 GHz | i7-8850H | 16 GB | 512 GB SSD | Radeon Pro 560X | grigio siderale | US | 747 € | 30 giorni di prova gratuita
Apple MacBook Pro with Touch Bar review: Second-screen dream machine - CNET
MacBook Pro con Touch Bar: il primo modello è un prodotto vintage
Apple Macbook pro 16" touch bar (configurato) HomePage - | Acquista Online
MacBook Pro: perché Apple dice addio alla Touch Bar...
New leak claims the Touch Bar isn't dead after all | Macworld
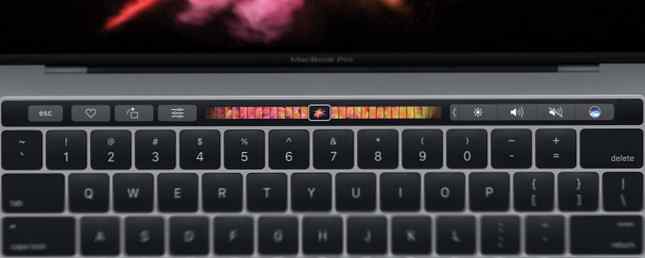
10 app che fanno buon uso della Touch Bar di MacBook Pro / Mac | Notizie dal mondo della tecnologia moderna!
MacBook Pro 13” TOUCH BAR 2016 (MOD A1706) | Riderstore
Apple MacBook Pro 2016 | 13.3" | Touch Bar | 2.9 GHz | 8 GB | 256 GB SSD | grigio siderale | IT | 569 € | 30 giorni di prova gratuita
